Half Life formula
What is Half Life ?
“The Time during which half of the unstable radioactive nuclei disintegrate is called the half-life of the sample of radioactive element.” A half-life formula is also provided here.
Keep reading…
We have seen that whenever an α or β-particle is emitted from a radioactive element, it is transformed into some other element. This radioactive decay process is quite random and is not subjected to any symmetry. This means that we cannot foretell about any particular atom as to when will it decay. It could decay immediately or it may remain unchanged for millions of years. Thus we cannot say anything about the life of any particular atom of a radioactive element.
Let us take the example of a city with a population of one million and we knew that on average ten-person die every day. Even with this knowledge, we cannot say with certainty which particular person will die on which particular day. We can only say that on the whole ten-person will die. The greater the population of the city, the greater the accuracy of such predictions.
As the population of a city, it is not possible to talk about an atom of a radioactive element. For more accurate results we always talk about large groups of atoms and laws of statistics that are applied to them. Let us suppose that we bring a group of 100,000 atoms under consideration and wait till such time that half of these i.e., 50,000 decay into their daughter element. This time is called the half-life T1/2 of this element.
See Also: Radioactive Decay
Half-Life Formula
If the half-life of the said element is one day, then after one day only 25,000 atoms will remain behind. That is with the passage of everyone’s day, the number of atoms remaining behind becomes half of the number already present.
Besides getting the definition of half-life we can deduce two other conclusions from this example. These are, firstly no radioactive element can completely decay. It is due to the reason that in any half-life period only half of the nuclei decay and in this way an infinite time is required for all the atoms to decay.
Secondly, the number of atoms decaying in a particular period is proportional to the number of atoms present at the beginning of the period. If the number of atoms to start with is large then a large number of atoms will decay in the period and if the number of atoms present in the beginning is small then fewer atoms will decay.
We can represent these results with an equation. If at any particular time the number of radioactive atoms is N. Then in an interval Δt, the number of the decaying atom, ΔN is proportional to the time interval Δt and the number of atoms N, i.e.,
ΔN ∝ – NΔt
or ΔN = – λ N Δt ………..(1)
Where λ is the constant of the proportionality and is called decay constant. In equation shows that if the decay constant of any element is large then in a particular interval more of its atoms will decay and if the constant λ is small then in that very interval less number of atoms will decay. From Eq (1) we can define decay constant λ as given below:
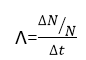
here ΔN/N is the fraction of the decaying atoms. Thus decay constant of any element is equal to the fraction of the decaying atom per unit of time. The unit of the decay constant is s -1. The negative sign in equation (1) indicates the decreases in the number of atoms N.
The decay ability of any radioactive element can be shown by a graphic method also:
We knew that every radioactive element decay at a particular rate with time. If we draw a graph between the number of atoms in the sample of the radioactive elements present at different times and the time then a curve will be obtained. This graph shows that in the beginning, the number of atoms present in the sample of the radioactive element was N°, with the passage of time the number of these atoms decreased due to their decay. This graph is called the decay curve.
Read Also: Uses of Radioisotopes
How to calculate Half-Life?
After a period of one half-life, N0 / 2 number of atoms of this radioactive element is left behind. If the wait is further for another half-period then half of the remaining N0 /2 atoms decay and 1/2 × No /2 = (1/2)2 No atoms remain behind.
After the expiry of a further period of a half-life, half of the remaining (1/2)2 N° atoms decay. The number of atoms that remain un-decayed is 1/2 × (1/2)2 No = ((1/2)3 No.
We can conclude from this example that if we have N° number of any radioactive element then after a period of n half-lives the number of atoms behind is (1/2)n No.
It has been found that the estimate of decay of every radioactive element is according to the graph but the half-life of every radioactive element is different.
For example, the half-life of uranium-238 is 4.5 ×109 years while the half of radium-226 is 1620 years. The half-life of some radioactive elements is very small, for example, the half-life of radon gas is 3.8 days and that of uranium-239 is 23.5 minutes.
From the above discussion, it is found that the estimate of any radioactive element can be made from its half-life or by determining its decay constant λ. It can be proved with the help of calculus that the following relations exist between the decay constant λ and the half-life T 1/2.
λ T 1/2 = 0.693 ….. (2)
Equation (2) shows that if the decay constant λ of any radioactive element is known, its half-life can be found.
Any stable element, besides the naturally occurring radioactive element, can be made radioactive. For this very high-energy particles are bombarded on the stable element. This bombardment excites the nuclei and the nuclei after becoming unstable become radioactive elements. Such radioactive elements are called artificial radioactive elements.
Radioactive half-life formula and examples (video)
Recent Posts
Is energy quantized in classical physics?
No, according to classical wave theory the emission of electromagnetic radiations from the surface is…
Types of laser
Basically, there are four types of laser which includes: Gas Lasers Solid State lasers Liquid…
Ultrasound frequency range
What is ultrasonics? The study and application of mechanical vibrations with frequencies beyond the limits…
Electromagnetic Energy: What are some examples of it?
Electromagnetic energy definition Electromagnetic energy is the amount of energy stored in a region of…
Fundamental units and Derived Units with Examples
The Main Difference between fundamental Units and Base units is that Units that Express base…
Newton’s First law of Motion Examples in Our Daily Life
Newton's first law of motion states that " A body continues its state of rest…