Terminal velocity examples

When a magnitude of the drag force becomes equal to the weight, the acting force acting on the droplet is zero. Then the droplet will fall with a constant speed called terminal velocity. A person falling from a certain height with constant speed is the terminal velocity examples.

Before we going to discuss terminal velocity, we will first shortly explain viscosity and Stokes law.

Keep reading…

What is viscosity?

“The fractional effect between different layers of a flowing fluid is known as viscosity.”

Viscosity measures, how much force is required to slide one layer of the liquid over another layer. Substances that do not flow easily, such as thick tar and honey, etc; have large coefficients of viscosity, usually denoted by the Greek letter ‘η’.Substances that flow easily, like water, have small coefficients of viscosity.

Since liquids and gases have non zero viscosity, a force is required if an object is to be moved through them. Even the small viscosity of the air causes a large retarding force on a car as it travels at high speed.

If you stick out your hand of the window of a fast-moving car, you can easily recognize that considerable force has to be exerted on your hand to move it through the air. These are typical examples of the following fact.

Table of the viscosity of liquids and gases at 30 C°.

Material	Viscosity 10-3 (Nsm-2)
Air	0.019
Acetone	0.295
Methanol	0.510
Benzene	0.564
Water	0.801
Ethanol	1.000
Plasma	1.6
Glycerin	6.29

What is drag force?

“An object moving through fluid experiences a retarding force called a drag force.” The drag force increases as the speed of the object increases.

Stokes law

“If drag force is directly proportional to velocity is called stokes law”.

F_D    ∝ V

The drag force F on a sphere of radius r moving slowly with speed v through a fluid of viscosity η is given by stokes law as under:

F = 6πηrV

At high speeds the force is no longer simply proportional to speed.

Stokes law derivation

Consider a water droplet such as that of fog falling vertically, the air drag on the water droplet increases with speed. The droplet accelerates rapidly under the overpowering force of gravity which pulls the droplet downward. However, the upward drag force on it increases as the speed of the droplet increases. The net force of the droplet is:

Net force = Weight – Drag force ………………(1)

As the speed of the droplet continues to increase, the drag force eventually approaches the weight in the magnitude. To find the terminal velocity v ₁ in this case, we use Stokes Law for the drag force. Equating it to the weight of the drop, we have:

From equation (1):

F = mg – F_D

F =mg – 6 πηrV_t

ma =mg – 6 πηrV_t

If m=0 then:

mg = 6 πηrV_t

V_{t =} mg/ 6 πηr  ………….(2)

By substituting the  mass of the droplet  ρV and volume  in equation (2) we get:

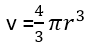
Read also:

Fluid mechanics

Bernoulli’s equation