Projectile motion equations

What is Projectile Motion?

Projectile motion is a type of motion experienced by an object that is projected near the Earth’s surface and moves along a curved path under the action of the force of gravity only if the effects of air resistance are assumed to be neglected. This curved path was shown by Galileo to be a parabola. The study of such types of motion is called ballistics, and this type of trajectory is a ballistic trajectory.

Projectile Motion Examples

• A bomb released from an airplane in level flight
• A bullet fired from a gun
• An arrow released from the bow
• A Javelin is thrown by an athlete

Projectile motion Equations

Up till now, we have been studying the motion of a particle along a straight line i.e motion in one dimension. Now we consider the motion of the ball when it is thrown horizontally from a certain height. It is observed that the ball travels forward as well as falls downwards until it strikes something.

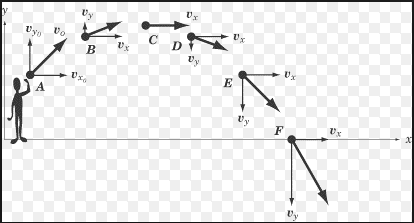Suppose that the ball leaves the hand of the thrower at point A and that its velocity at that instant is completely horizontal. Let this velocity be V_x .

According to Newton’s first law of motion, there will be no acceleration in the horizontal direction, unless a horizontally directed force acts on the ball. Ignoring the air friction, the only force acting on the ball during flight is the force of gravity.

There is no horizontal force acting on it. So its horizontal velocity will remain unchanged and will be V_x until the ball hits something. The horizontal motion of the ball is simple. The ball moves with a constant horizontal velocity component. Hence horizontal distance X is given by:

X = V_x×  t

The vertical motion of the ball is also not complicated. It will accelerate downward under the force of gravity and hence a = g . This vertical motion is the same as for a freely falling body. Since initial velocity is zero, hence, vertical distance y by using the second equation of motion is given by:

It is not necessary that an object should be thrown with some initial velocity in the horizontal direction. A football kicked off by a player; a ball thrown by a cricketer and a missile fired from a launching pad, all projected at some angles with the horizontal, are called projectiles.

Projectile velocity formula

In such cases, the motion of a projectile can be studied easily by resolving it into horizontal and vertical components that are independent of each other. Suppose that a projectile is fired in a direction angle θ with the horizontal by velocity V along the horizontal and vertical components of velocity V along the horizontal and vertical directions be V cosθ and V sinθ respectively.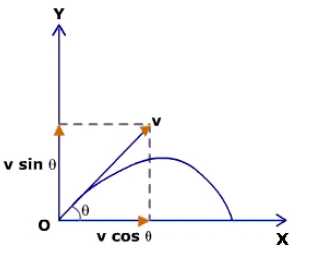

The horizontal acceleration is ax = 0 because we have neglected air resistance and no other force is acting along with the direction resistance and no other force is acting along this direction whereas vertical acceleration a_y = g. Hence, the horizontal component V_x remains constant and at any time t, we have:

V_{fx =} V_{ix =} V_icosθ

Now we consider the vertical motion. The initial vertical component of the velocity is V sinθ in the upward direction. By using the first equation of motion (V_{f =} V_{i +} at) the vertical component V_fy of the velocity at any instant t is given by:

V_{fy = Vsinθ  – gt}

The magnitude of velocity at any instant is:

V = square root of (V_{fx^{2 +}} V_{fv^{2 )}}

The angle Φ which this resultant velocity makes with the horizontal can be found from:

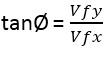
In P motion one may wish to determine the height to which the projectile rises, the time of flight and horizontal range.

Maximum height

The maximum vertical distance attained by the projectile is called maximum height. It is denoted by H.
Derivation for maximum height:

2 as =V_f ² –  V_i²

As the body moves upward, so a = -g , the initial vertical velocity V_{iy =}V_{i sinθ} and V_fy =0 because the body comes to rest after reaching the highest point. Since:

S = height = h

-2gh= 0 -V_i ²sin²θ

h=Vsin²θ/2g

Time of flight formula

“It is the total time for which the projectile remains in the air.” or The time taken by the body to cover the distance from the place of its projection to the place where it hits the ground at the same level is called the time of flight.
This can be obtained by taking S =h  =0, because the body goes up and comes back to the same level, thus covering no vertical distance. If the body is projecting with velocity V making angle θ with a horizontal, then its vertical component will be _Visinθ. Hence the equation is:

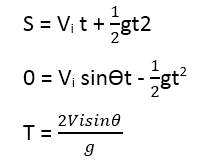
Range of projectile formula derivation

The maximum distance that a projectile covers in the horizontal direction is called the range of the projectile. Or The horizontal distance covered by the projectile between the point of projection and point of return to the level of projection is called the “Range” of the projectile and is represented by “R”.
To determine the range R of the projectile, we multiply the horizontal component of the velocity of projection with the total time taken by the body after leaving the point of projection.
Thus the range of the projectile depends upon the velocity of projection and the angle of projection.
Therefore:

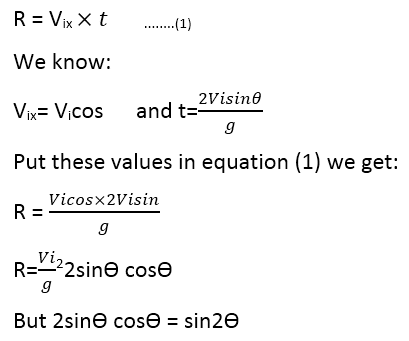
Thus
R = Vi²Sin2θ/g
For the range R to be maximum, the factor sin2θ should have a maximum value which is 1 when 2θ =90° or θ=45º
R= Vi²/g

Types of projectile motion

Common Types of Projectile motion are given in the list below:

• Kinetic projectiles
• Sports projectiles
• Delivery projectiles
• Wire projectiles

watch also the video:

Related Topics:

• Types of motion
• Newton’s First law of motion
• Equations of motion
• Newton’s Second law of motion

External sources:

• http://www.physicsclassroom.com/class/vectors/u3l2a.cfm
• https://en.wikipedia.org/wiki/Projectile_motion