Viscosity : Definition, formula, Examples and applications

In this post, You’ll learn Examples of viscosity in everyday Life.
So, If you want to get benefits from this post you’ll love this post.
Let’s dive right in:
Contents:

• Definition of Viscosity
• Viscosity formula
• Viscosity Examples
• Applications
• Much more

What is Viscosity?

“Viscosity is the  internal friction in a fluid.”Viscous forces oppose the motion of one portion of a fluid relative to another. Viscosity is the reason it takes effort to paddle a canoe through calm water, but it is also the reason the paddle works. Viscous effects are important in the flow of fluids in pipes, the flow of blood, the lubrication of engine parts, and many other situations.

Fluids that flow readily, such as water or gasoline, have smaller viscosities that do ‘thick’ liquids such as honey or motor oil. Viscosities of all fluids are strongly temperature-dependent, increasing for gases and decreasing for liquids as the temperature increases.

Friction is a phenomenon that takes place in all of reality. A frictional force comes into existence whenever any two solid bodies in contact are in relative motion. It is a consequence of the adhesive forces between the surface molecules of the bodies in contact. At a fundamental level, it is thought to be of a mutually induced dipole interaction and is quite complex. The basic principles of frictional forces have been derived from empirical studies and the laws have been applied to most of all practical examples. In fact, tribology is a very important topic of consideration in the modern industry with potent economic consequences.

When an opposing force of the nature of frictional force occurs between fluid layers in a moving fluid is called ‘viscosity’. This viscosity is not considered in a derivation of Bernoulli’s principle but it is a necessary prerequisite for the establishment of laminar flow that is assumed in its derivation.

Coefficient of viscosity

Newton, on the basis of experimental observations, gave the expression for the viscous force:

For an incompressible fluid in steady streamlined flow, the force is proportional to A, The surface area between layers in contact, and the velocity gradient is along the direction normal to the laminar flow. The coefficient of viscosity is given as Nsm^-2 or as Pa s or in SI units as Poiseulles, after the early investigator of this phenomenon.

It turns out that the coefficient of viscosity is given by:

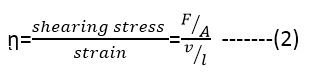
By the virtue of the above perspective, Maxwell considered a liquid to possess a certain amount of rigidity that continually resists for fleeting moments and hence he termed it as a ‘fugitive elasticity’.
See Also: Terminal Velocity

Critical velocity and Reynolds number

A liquid inflow, more particularly through a tube, may experience streamlined or laminar flow as the velocity is increased, till a certain value, the critical value, v_c at which the steady flow is disturbed. There is no more laminar flow and flow lines of different layers shall cross each other. The liquid flow could then have eddies and great turbulence. Osborne Reynolds demonstrated that the critical velocity of a liquid is given by a relation of the form:

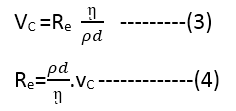
Where is the coefficient of viscosity and is the density of the liquid, d is the diameter of the tube carrying the fluid? ’R_e’ is called Reynold’s number and from a dimensional analysis of the equation, we see that it is just a number. ’d’ may be taken to stand for the typical dimension of the obstacle or boundary to fluid flow.

In the case of an obstacle like a sphere, for instance, it could be the diameter of the sphere and for flow through a tube, it could be the diameter of the tube. This law has passed several rigorous experimental tests and found to be valid. It is a much-used critical number that looks like the ratio of the inertial force per unit area to the viscous per unit area.

See More: Continuity equation

Applications of viscosity

In the case of motion of bodies through viscous media, the number obeys the ‘law of similarity’. Hence, geometrically similar bodies have similar streamlined flow in the same liquid. The technical importance of this number is that the resistance of a medium to the motion of the body, of any shape, may be ascertained by using a scaled-down model under lab conditions, with the same liquid.

The experimental results may be assumed to hold for the real situation also. Geometrically, similar flows become turbulent at the same values of R_e for the different combinations of and v_C. For example, if oil and water are pumped through tubes of identical shapes and cross-sections, the flow becomes turbulent at approximately the same value of R_e, for the suitable combinations.

When the critical velocity is exceeded in any situation, turbulence sets in and this leads to energy dissipation, mostly as heat. Turbulence results in the rapid mixing of composition and energy of the medium. This aspect is used for benefit in certain cases where it may be needed, like, in the kitchen mixie. Weather in parts of the world is conditioned by ocean currents of large proportions. Turbulence manifests as noise in blood flow through arteries, where it enables blood pressure measurement.

Scaling and the law of similarity help in the design of vehicles such as cars, planes, and other fast-moving vehicles so that the smooth motion of the vehicles is not disturbed by the turbulence in the medium, in which they move.

For the flow of liquids in tubes, the characteristics of Reynolds number values are:

Viscous Drag and terminal velocity:

The viscous drag experienced by any object in a viscous medium is seen to be proportional to the velocity of the body in the fluid. Stokes discovered the expression for retarding force an object moving in a medium for viscosity :

Where a is the radius of the sphere and v is the velocity of motion of the body.

Let us suppose that an object is moving in a viscous liquid under the force of gravity. Under the acceleration, the body’s velocity keeps on increasing and from equation (5), consequently, the retarding force keeps increasing. At a certain point in its motion, the force on the body would cancel out and the body suffers no further change in velocity. This ‘terminal’ velocity in the motion of the falling body in a viscous medium had been used by stokes to determine the viscosity of highly viscous liquids in the laboratory.

You need not look further for the benefits of this natural phenomenon. Water drops fall from rain-bearing clouds that are found to form in heights ranging from 2.5 to 6 km. The typical size of these drops could vary from 0.05 to 0.5 cm in radius. For a trial calculation, let us assume a drop of 0.4cm radius coming down under the influence of gravity. From the fundamental equations of dynamics, we know that:

V² =  u² + 2gs

And for u=0 ,g=9.8ms^-2,and s=4000 m, let us say, the drop would gain a velocity of:
V²= 2 ×9.8×4000
V=280 m/s.

This would be a frighteningly enormous value and could prove to be a fatal bullet if it strikes us. But we do know that the typical velocity of such a drop would be 9 m/s. This manageable high value of the terminal velocity. The evolution of nature and all its parts that allowed life to exist on this earth is a mysterious truth and a scientific reality. If the protective canopy of the atmosphere was not there, even rainfall could destroy life on earth.

See Also: Pascal law

Poiseuille’s law

For liquid flow through a capillary tube, gives the coefficient of viscosity of the liquids as:

Where V is the volume of liquid flowing through the capillary per unit time, P is the pressure difference between the two ends of the tube, r is the radius of the capillary tube and l is its length.

In turbulent flow, the flow rate is not directly proportional to the pressure gradient, as in laminar flow. Using the definition of the Reynolds number eq (4), we can see that when the density of the blood is high, for a large diameter with the rapid flow there is a tendency towards turbulence. When a narrow vessel widens to a larger one and the blood flow experiences such changes rapidly, turbulence may result in a characteristic noise. An atheroma may be the cause of turbulent flow. A skillful medical practitioner may just need a stethoscope to detect signs of such a condition by noting any such turbulence.

Zero viscosity –Superfluids

Super-fluidity is a quantum phenomenon that may be observed in materials that exist in the liquid state at very low temperatures. As helium is cooled to 2.17k, a characteristic discontinuity occurs in the heat capacity curve and part of the He ‘condenses’ to its ground state in what is called the Bose-Einstein condensation.

The liquid density drops, and this part of the liquid does not contribute to the heat capacity while also becomes a superfluid with zero viscosity. Superfluidity would mean that the liquid would creep up vessels, or slip out easily through microscopic pores in what seemed to be vacuum-tight containers. If a capillary tube is inserted into the liquid, the liquid would climb up the capillary and squirt like a fountain out of the tube.

Viscosity (video)

Related Topics:

• Mechanics