Moment of inertia: Definition, formulas & Equation
In this post, You’ll Learn a List of the moment of inertia formulas for Different Shapes with examples.
Contents:
- Moments of inertia Definition
- The formula of the moment of inertia
- Equation
- Unit
- Much more
Keep reading…
What is moment of inertia?
Moment of inertia ( I ) is defined as The sum of the products of the mass of each particle of the body and square of its perpendicular distance from the axis. It is also known as rotational inertia. The moment of inertia reflects the mass distribution of a body or a system of rotating particles, with respect to an axis of rotation. The moment of inertia only depends on the geometry of the body and the position of the axis of rotation, but it does not depend on the forces involved in the movement.
The moment of inertia reflects the mass distribution of a body or a system of rotating particles, with respect to an axis of rotation. The moment of inertia only depends on the geometry of the body and the position of the axis of rotation, but it does not depend on the forces involved in the movement.
The moment of inertia plays a role analogous to that of the inertial mass in the case of rectilinear and uniform motion. It is the scalar value of the longitudinal angular momentum of a rigid solid.
I = mr²
For a rigid body moving about a fixed axis, the laws of motion have the same form as those of rectilinear motion, with the moment of inertia replacing mass, angular replacing linear velocity, angular momentum replacing linear momentum, etc. Hence the kinetic energy of a body rotating about a fixed axis with angular velocity ω is ½ω², which corresponds to ½mv² for the kinetic energy of a body of mass m translated with velocity v.See also Routh’s rule; the theorem of parallel axes.
Moment of inertia equation
Consider a mass m attached to the end of a massless rod. Let us assume that the bearing at the pivot point O is frictionless. Let the system be in a horizontal plane. A force F is acting on the mass perpendicular to the rod and hence this will accelerate the mass according to:
F = ma
In doing so the force will cause the mass to rotate about O.Since tangential acceleration is related to angular
acceleration α by the equation.
angular acceleration = rα
As the turning effect is produced by torque τ, it would, therefore, be better to write the equation for rotation in terms of torque. This can be done by multiplying both sides of the above equation by r. Thus
rF = τ = torque = mr²α
Which is the rotational analog of Newton’s second law of motion?
Here F is replaced by τ, a by α and m by mr². The quantity mr² is known as the moment of inertia and is represented by I.
Importance of moment of inertia
The moment of inertia plays the same role in angular motion as the mass in linear motion. It may be noted that moment of inertia depends not only on mass m but also on r².
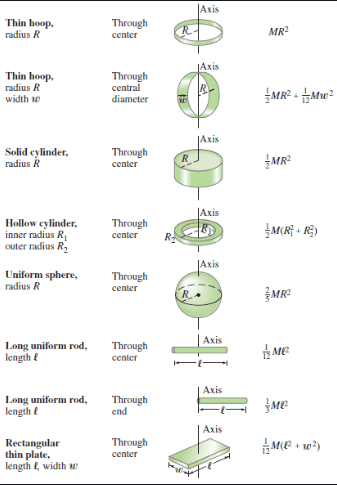
Moment of inertia Formulas
Here’s the list of formulas of the moment of inertia of Different shapes:
-
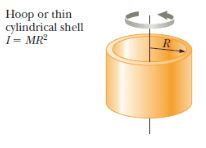
Moment of inertia of Hoop
-
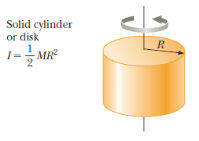
Moment of inertia of a disc
-
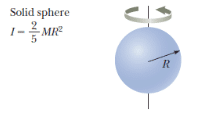
Moment of inertia of a solid sphere
-
Moment of inertia of a hollow cylinder
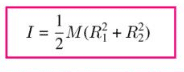
-
Moment of inertia of thin rod

-
Moment of inertia of a rectangle

-
Moment of inertia of a long thin rod

-
Moment of inertia of a spherical shell
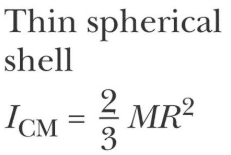
Anyone have a definition or conversion to inch pounds squared for moments of inertia in mugs???